Okay, I’m going to classify this article as Random Bulls$&%. Let’s start with that. Because this article is kind of a departure from what I usually do. But there’s a couple of reasons to post it. This article is actually about understanding some basic stuff about probability and random chance and dice and ultimately it uses a few questions about how games work to illustrate some of the basic concepts of probability.

Doesn’t that sound like fun? I mean, everyone loves math, right?!

No?

Okay, I’m going to level with you. Probability math is something that most GMs don’t need to bother with. I mean, it’s good to understand it, but you really don’t HAVE TO. At least, you don’t have to unless you’re going to f$&% with your game. And, in a little while, I’m going to give you a great example of a PROFESSIONAL GAME DESIGNER attempting to f$&% with his game and not realizing the damage a seemingly simple change can do.

This is technical, mechanical minutia. I can’t lie about that. And I can’t pretend it it’s important to every GM and every game. For example, if you’re happy with your story-gamey FATE bulls$&% or whatever or you think the rules are just there to get in the way of the fun, this article ISN’T for you. But if you believe the rules serve a purpose and that purpose is to define the likelihood of success and failure, then this s$&% becomes super important. Because probability math becomes the tool by which you can tweak your probabilities of success.

For example, let’s say you’re designing a game and you invent a dice mechanic. Neat, right? But now, here’s the important question: how often should the PCs succeed at a thing. Given any random thing that they could do in the game, how likely should success be? How often, for example, should an attack roll actually hit? Half the time? Two thirds? Three quarters? Every game is different and every game designer and player is a little bit different, so the qualitative answers vary. D&D, for example, seems to say that about two-thirds of all die rolls should be successes. And, based on its popularity and the lack of complaints, that seems to be okay for most people. So, D&D took the dice mechanic and decided that, given all of the bonuses and difficulty numbers, roughly speaking, an average PC should succeed at a moderate difficulty task about 70% of the time. Well, that has varied a little bit over time, to be honest. In 3E and 4E it was about 65%. Near as I can tell, it’s 70% in 5E.

Now, if you’re not a game designer, you might figure you’ll never be in a situation where you have to worry about that. And that MIGHT be true. But, as a GM, you are constantly asked to make minor game design decisions. You have to set difficulties and provide bonuses and penalties. That’s part of your job. Now, sure, the games provide guidelines for doing that. And you can just follow those. But what happens when you want to deviate from those? How is it going to affect your game? And what if you want to add a new rule? For example, suppose you’re playing Pathfinder or D&D 3.5. And you want to add an “instant kill critical” rule. Normally, in those games, if you roll a natural 20, you MIGHT score a critical hit. You have to roll the attack again. If the second attack hits, it really is a critical. But you can add a rule that says, if you roll a 20 again, you instantly slay the thing you’re attacking. What impact is that going to have on the game? How do you work that out?

Look, long story short: if you want to skip this article because it’s math and probability and game design, fine. Do it. Sorry it isn’t for you. But I really think this stuff IS worth knowing because it empowers you to tinker under the hood of your game and understand how things are really working.

The Mystery of Advantage and Where This Article Came From

Let’s talk about D&D 5E. In D&D, everything is determined by an ability check. Roll 1d20, add an ability score modifier, add other relevant bonuses, and compare the result to a difficulty number. If the modified roll equals or exceeds the difficulty, the thing works. Otherwise, the thing fails. It’s pretty simple. And because it’s pretty simple, if you have a basic understanding of probability, you can figure out how likely success is given the modifiers and the difficulty. But 5E throws a couple of monkey wrenches into that plan. It throws in advantage and disadvantage. Sometimes, you have advantage on a die roll. That means you roll the d20 TWICE and take the better result. Other times, you have disadvantage. That means you roll the d20 TWICE and take the worse result.

In days gone by – that is the 3E, Pathfinder, and 4E days – we didn’t f$&% around like that. We just added modifiers. And modifiers were easy. Again, if you knew the basic probability math, you knew how much a modifier would affect the chance of success or failure. And that was because d20s provide a nice LINEAR probability. But rolling two dice and taking the higher roll makes a complete mess of things. Now, because I understand probability math, I was aware that, roughly speaking, advantage and disadvantage were usually the equivalent of a +4 or -4 under the old system. But I knew that was very fuzzy. And I found myself wanting to work out the actual probabilities and understand how advantage and disadvantage really affected the system.

I started doing the math and I tweeted about my results a bit on Twitter and people were intrigued because I told them a few things that they didn’t realize. For example, advantage and disadvantage have the greatest effect if the number you NEED on the d20 is between 8 and 14. For results higher or lower than that, advantage and disadvantage had smaller and smaller effects, to the point where, if you were looking for a 20 on that d20, advantage was practically useless. People asked why. Sadly, it’s just not the sort of thing you can explain on Twitter.

At the same time, I was working through another probability problem. I wanted to know this: assume two people are rolling dice against each other. Each has a die ranging from d4 to d12. Higher rolls wins. What are the odds of a player winning given any combination of dice.

Actually, the question is more complicated than that. But I can’t talk about a lot of it because of trade secrets and NDAs and other stuff. So don’t ask.

And THAT reminded me of something that happened with a game designer a few years ago. This game designer – a professional designer who worked for Wizards of the Coast at the time who I will not name – publicly suggested on Twitter that you could replace the d20 roll in D&D with a roll of 3d6 and have a minimal impact on the game as long as you treated the result of 6,6,6 as a crit. And a few of us who knew our probability math proceeded to tell the designer why that was dumb and wrong.

And THEN, a few people started asking me about the difference between rolling 3d6 for ability scores and rolling 4d6 and dropping the lowest.

The end result was I talked a lot of probability and statistics on Twitter for a couple of days and a lot of people told me there’d be interest in an article about it. And then I did a poll about whether people wanted this article and learned that a lot of people do. So. Here we are. This article.

And here’s what we’re going to do. We’re going to discuss some BASIC concepts (and misconceptions) about probability and dice in RPGs and then we’re going to explore some of the specific questions I mentioned above as a way of digging into the complexity of probability.

You’re f$&%ing welcome.

A Basic Introduction to Probability

The mathematics of probability are a subset of the branch of math called statistics. And statistics deals with the collection, analysis, and interpretation of data. The whole point is to reach useful conclusions or to predict future events. For example, if we analyze crime data and discover that sixty percent of all violent crimes happen in a certain neighborhood of our city, we can focus more of our police efforts in that neighborhood. That’s a very simplified conclusion, mind you. And in real life, it’s probably not a good conclusion without more information, but it just demonstrates the point of the study of statistics.

The problem is that people misunderstand and misuse statistics. And this is something that even people in role-playing games do. I’ll give you a very relevant example. In America, the average height of a man is 5’ 9”. The average height of a woman is 5’ 4”. If you’ve ever been to a theme park, you know that certain intense rides – like roller coasters – often have a minimum height requirement because of the way the harness, seats, and safety devices are designed. If you’re below the minimum height, your neck might snap like a dry twig on a sharp curve because the harness isn’t supporting you properly.

Now, if you pick out any random man and woman in America, you would expect the woman to be 5” shorter than the man. That makes sense, right? That’s how statistical analysis works.

Now, imagine you are a woman who is 5’ 6”. You go a theme park and you find a roller coaster whose minimum height is 5’ 5”. You figure, neat, I can ride this. But then the theme park employee stops you. “Sorry,” he says, “you can’t ride this ride. The minimum height is 5’ 5” and the average woman is only 5’ 4” tall.” So, you punch him and ride the ride anyway because he’s a moron.

The point is statistical analysis and probability let you make some guesses about what is likely and what isn’t and they let you make broad statements about a population. But any given individual result is still down to random chance. And you can’t use statistical tools on individuals. Which is something morons who scream about privilege need to understand. But I digress.

In essence, probability about determining the chance of a given outcome of an event. But that probability doesn’t erase the fact that the event is random. You might have a 95% chance of hitting the goblin on your next attack roll, but that doesn’t mean you can’t roll a 1. And it might be very unlikely, but you can roll three 1s in a row.

You can design a system wherein the players will succeed on 80% of their actions, but any given action might succeed and it might fail and any player might get a run of really bad or really good luck. The outcomes are still random.

When an individual player talks about probabilities, they usually want to know the chance of getting a specific outcome. What’s the chance I’ll roll a 15 for my Strength score if I roll 3d6? If I roll 4d6? What’s the chance I’ll get a 10 or above? What are the chances I’ll manage to kill this goblin this round? And the problem with those questions is that they are talking about a SINGLE EVENT.

When game designers talk about probabilities, they want to know how lots and lots of die rolls are going to come out. If I make my system work like this, how often will the players succeed? How likely is this rule to kill a PC? If the PCs have this stat and the monsters have that stat, how often will the PCs win a fight? And THOSE questions are what probability was really meant to do.

It comes down to this thing called the law of averages. So let’s look some math and understand that.

The Chance of a Thing Happening

Let’s start with the most basic probability rule there is. What’s the chance of something happening? Imagine you roll a d6. What’s the chance you roll a 6? The easiest way to compute the probability of an event is to figure out how many different outcomes there are. Next, figure out how many of those outcomes are the one you want. Divide the number you want by the total number and, BAM, you have probability.

So, what’s the chance of a 6 on a d6? Well, there’s six different numbers on a d6: 1, 2, 3, 4, 5, and 6. Hope we didn’t blow any minds there. But only one of them is the one we want: 6. So, 1 outcome we want divided by 6 total outcomes equals 1/6 or 0.1667 or 16.7%.

The thing is, we can express probabilities in lots of different ways. We can say “there is a 1 in 6 chance of of rolling a 6 on a d6.” We can express that as a fraction: 1/6. Or we can divide out the fraction and get 0.1667. And we can multiply that decimal by 100 to get a percentage. Now, most math nuts work with decimals because they make the math easy. But most normal people find percentages to be the most intuitive. So I’m going to stick with percentages.

A percent, by the way, comes from the Latin for “out of one hundred.” So, if we roll the d6 one hundred separate times, we would expect 6 to come up about 16 times. 16% means we expect the result 16 times out of every 100 attempts.

Let’s try a harder one. What are the chances of rolling 4 or better on a d8? Try it yourself.

There’s 8 total outcomes, right? 1 through 8. But only five of them are the ones we want: 4, 5, 6, 7, and 8. So, there’s a 5 in 8 chance. 5 divided by 8 is 0.625 which is 62.5%.

Did you guess 50%? Hahaha. Well, I did that on purpose to illustrate something that comes up a lot with dice in RPGs. Because we’re always trying to roll a specific number OR HIGHER, the probabilities tend to run slightly higher than we intuitively guess. Always be careful to actually count the outcomes so that you don’t get tripped up.

What We Expect

And that brings us around to something we call the expected value. Some folks call this the average. Some folks call this the mean. And, if you want to get technical, there is a difference between average, mean, and expected value. But when we talk like game designers, mean, average, and expected value generally mean the same thing. The trouble is, game designers and gamers alike REALLY misuse the expected value. So let’s define it carefully.

First of all, let’s talk about the average. The average, in math, is the middle of all of the results. If you take all the results of something and write them down, there’s a midpoint. Half the results are above that point and half the results are below that point. That’s the average. So, for example, when we say that the average height of a man in the US is 5’ 9” tall, what we mean is that if you took ALL of the men in the US and lined them up in height order, the person in the middle of the line would be 5’ 9”.

Now, let’s say you roll a d6 three times. And you get 4, 4, and 6. We compute the average by adding up all the results and dividing by the number of results. And that tells us the average is 4.7. The average of THOSE THREE SPECIFIC ROLLS that is. As game designers and game masters, we generally don’t care about what we call a SAMPLE AVERAGE – the average of a few specific outcomes. We care about the real average. If we were going to roll the die an infinite number of times, what result would be in the middle? And with a d6, you find the answer is 3.5. And that makes perfect sense. If you roll a die an infinite number of times, you’d expect half the time to get 1, 2, or 3; and the rest to get 4, 5, or 6. Right? 3.5 is the number in the middle of all the results.

It is also impossible to roll. Try as you might, you will never actually roll a 3.5. Go ahead. Try.

When you take the average over infinity, you get something called the expected value. And, often, when you’re doing game design math, you assume that the expected value is the one that is going to come up on any given roll. That’s why, for example, instead of rolling hit points in 5E, we simply assume you rolled a little over half your hit die. Because 2.5 is the expected value of a d4, 3.5 is the expected value on a d6, 4.5 is the expected value on a d8, and so on.

Just don’t confuse that. We don’t expect the “expected value” to be the one that comes up the most. It’s just the result that is in the exact middle of all the rolls ever rolled. It’s useful as an average, but there are much more useful tools. As we’ll see.

The Law of Averages

And that brings us to the law of averages. Roughly speaking, the law of averages states that the more times you do a thing, the closer the average result comes to the expected value. If you roll a d6 once, you could get any number. Roll it twice, the average could be anything. Three times, the average is still going to be wonky. But if you roll it a hundred times or a thousand or a million or a billion, the results are going to start matching the probabilities.

Just keep that s$&% in mind. Yeah, you’d expect one crit out of every twenty attack rolls, but in reality, the number of crits is going to be random and you might have three in ten rolls or none in thirty rolls. You have to roll an infinite number of attack rolls before you are guaranteed to see one crit in every twenty.

Certainty and Impossibility

It should be noted that, whether you use fractions or decimals, if you add up the probability of ALL possible events, you’ll get 1. And 1 translates to 100%. If something has a probability of 1 or 100%, that means its guaranteed to happen. What are the chances of rolling a 1 or better on a d6? 100%. Six outcomes and six that we want. 6 divided by 6 is 1.

On the other hand, a probability of 0 or 0 or 0% can’t ever happen. What are the chances of rolling a 7 or better on a d6? 0. Six outcomes and none of them good. 0 divided 6 is 0.

But knowing that allows to take a very useful shortcut in computing probabilities. And that is based on complements.

The Chance of Not a Thing

Here’s the deal. If you know the chance of a thing happening is 47%, what’s the chance of the thing not happening? That’s 53%, right? Because all the probabilities have to add up to 100%. This is an extremely useful trick because sometimes it’s easier to calculate the chance of something NOT happening than it is to calculate the chance of it happening. For example, what’s the chance of a 4 or better on a d30? Well, you could count all the results you want: 4, 5, 6, 7,… etc. Or you could figure that there’s only 3 outcomes you don’t want: 1, 2, and 3. So, 3 divided by 30 is 0.1 or 10%. That means the chance of a 4 or better is 90%.

Quick Review of Basic Probability

The probability of something happening is the total number of ways that thing can happen divided by the total number of things that could possibly happen. If you divide it out and multiply by 100, you get a percentage. The average result is the result that would be right in the middle if we made a list of all the results that actually happened. The expected result is just the average result of all the possible results multiplied by their probabilities. In other words, if we did the experiment infinity times and found the average, we’d have the expected value.

All the possible outcomes have to add up to 100% probability. So, if you know the chance of thing happening and you subtract that from 100%, you’ll get the chance of it not happening. And sometimes that’s easier.

Tricks with Weighted Averages and Expected Results

Now, we’ve talked about the average and we’ve talked about the expected result. And we’ve talked about probability. How does this help us design a game? Well, let’s look at one trick we can do with expected values.

So, we know that we can figure out the expected value of a given die roll, right? Let’s say a d8. If we roll a d8 infinity times, the average result is going to be 4.5. We simply add all the results (1+2+3+etc.) and divide by the total number of results (8). That tells us that, if people roll a d8 lots of times, sometimes the results will be above 4.5, sometimes below 4.5, but they will tend to even out at 4.5. For example, let’s say we have a weapon in our game like a longsword that does a d8 of damage. We can’t say much about individual outcomes, but across all the players in all the games, we can assume that each person doing a longsword will effectively do 4.5 damage with every hit.

And now we can do something called a weighted average. Let’s suppose, for example, we assume that a first level monster has an AC of 13 and a first level PC has a +5 on their attack roll. That’s actually what D&D 5E assumes (more or less). So that means that a PC has a 65% chance of hitting on an attack. Now, the same PC is probably going to have a damage bonus of +3. Which means their average damage with each hit will be 7.5 (4.5+3).
Now, here’s a neat trick called a weighted average. If you multiply the probability of a thing happening by the expected result if it DOES happen, you get a weighted average. In this case, for example, multiplying 65% (0.65) by 7.5 gives us 4.88. What does that mean? It means that while some fighters will miss, some will hit, some will roll high damage, and some will roll low damage, overall, all of the fighters rolling all of the attacks with all of the longswords can be expected to do 4.88 damage per round.

So, suppose you want monsters to be able to last for five rounds against a single fighter. You know your 1st level monsters need 25 HP to stand up to that punishment. That takes into account that some rounds, the fighter will miss, some they will hit and do lots of damage, and some they will hit and do light damage. A monster might only last for two or three rounds against a lucky fighter. Or against an unlucky fighter, a monster might stand up for eight rounds. But, overall, across the whole span of your game, monsters will stick around for five rounds.

Actually, weighted averages are even more powerful. I’ll give you an example by taking into account the thing we forgot in our damage calculation. Because you can multiply each outcome by its probability and add them all together, we can factor in critical hits. Let’s look at it like this.

35% of the time, the player will roll less than 8. And they will do 0 damage. 60% of the time, the player will roll anything between 8 and 19 (inclusive) and deal 7.5 damage. But 5% of the time, the player will roll a 20. And then they get a critical hit, rolling the damage die twice and adding the modifier. On a crit, they will do 4.5 + 4.5 + 3 or 12 damage (two rolls + 3 modifier).

35% x 0 damage = 0 damage.
60% x 7.5 damage = 4.5 damage.
5% x 12 damage = 0.6 damage.
0 + 4.5 + 0.6 = 5.1 damage.

That lets us play with thought experiments. Let’s say, for example, we decided that anything from 18 to 20 was a crit. How would that change the damage output? Well, that would 50% x 7.5 damage (3.8 damage) plus 15% x 12 damage (1.8 damage) or 5.6 damage. Not a big issue, is it? No, not really. But what if the crit also did triple damage. Or what if the modifier on the damage was also tripled. That can happen with certain combinations of feats and weapons in Pathfinder or 3.5. Now you have the power to figure that out.

See if you can work out what the odds would be assuming the same +5 to hit, 13 AC, and damage of 1d8+3 assuming a critical happens on a 20 and does 2d8+6. Then work out what it would be a critical happened on an 18 to 20 and did 3d8+9 damage.

Of course, Pathfinder and 3.5 also involve confirmation rolls. And in order to work those out, we need to know how probabilities combine.

Combining Probabilities

The last BASIC tool we need in our arsenal is to figure out how to combine probabilities. For example, let’s say you need at least a 12 to hit the goblin on a d20. And the goblin has 4 hit points left. You roll a d8 for damage. What’s the chance you’re going to kill the goblin if you attack?

Well, to kill the goblin, you need a 12 or better on a d20. And you need a 4 or better on a d8. We can figure those two probabilities independently. 45% on the attack and 62.5% on the damage. But how to combine them into one super probability.

When two events are independent – that means each one happens on its own without affecting the other – we can figure out the probability of both things happening by multiplying them. Most things in RPGs are actually independent of each other. The d20 doesn’t affect the d8. 0.45 times 0.625 equals 0.28125. So the chance of killing the goblin is 28.125%.

Now, sometimes we need to know the probability of getting one thing OR another. And this is where things actually get kind of confusing. But this is super important because it’s at the heart of understanding how advantage and disadvantage work. In fact, figuring out THAT answer will help us understand how this OR that probability works.

How Does Advantage Work?

Before we launch into how advantage works and understand how probabilities combine, it’s important to first understand something wonky about RPGs and probabilities. So, let’s talk about what you need on the die first.

What You Need on the Die

Suppose you are trying to pick a lock. It’s 5E D&D. You have a Dexterity modifier of +3. And because you are proficient with Thieves’ Tools, you get to add your proficiency bonus of +2. That means you have a total of +5. You’re going to roll a d20 and add 5. Right? Now, suppose the DC to pick the lock is 13.

When it comes to dealing with probability in RPGs, things seem really muddy because of modifiers. We’re always adding s$&% and subtracting s$&% and it’s a pain in the a$&, right? How does that affect the probability math? Honestly, it doesn’t. I mean, you can try to futz around with it. But the easiest way to handle it is just to figure out what you actually need on the die.

In the example above, you – the rogue – will succeed if you roll an 8 or better. Right? You need a 13. 8 plus 5 is 13. So, you’re concerned about the chance of rolling an 8. By the way, the chance of rolling an 8 or better on a d20? It works out to be 65%.

And, if you’ll allow me a little digression, that’s how we can figure out what the game designers thought was a good chance of success. Look at 5E for example. We know from PHB 174 that the average DC is 15. Because it’s a party based game, most PC parties are going to have one member with a +3 or +2 in whatever ability score is relevant to a task at hand. And because of the small number of skills and the way players tend not to overlap skills, those party members are also very likely to have proficiency bonuses for the relevant tasks, and those start at +2. So, most of the times, a given task is going to have a DC of 10 to 15 and the PC is going to have a +4 to +5. At least from 1st to 4th level. That means, the party will almost need either a 5 or better on the die or a 10 or better on the die. And thus, the chance of success for any task from 1st to 4th level is between 55% and 80%. If you use similar assumptions to follow the progression up through the levels, you’ll find that, over the course the game, it converges on around 70%.

But that aside, in this article, we’re always going to be shaving off all the modifiers and looking at what you actually need on the die.

How Does Advantage Work?

Okay, now: you’re a rogue with a total of +5 to pick locks facing a DC 13 lock. You need an 8 or better on the die. And that means you are 65% likely to succeed. But what if you have advantage? What if you get to roll 2d20 and keep the best result? What then?

What that question really boils down to is this: given two dice, what is the probability that you roll an 8 or better on the first die OR roll an 8 or better on the second die.

How can we go about solving this dilemma?

Normally, with probability questions, it helps to look at a simpler question first. And it also helps to use something called enumeration. So let’s invent a simpler problem. Let’s say you flip two coins. What are the odds of getting at least one that lands on heads?

Enumeration is the act of listing all of the possible results. So, you might get HH, HT, TH, and TT. Four results, three are the ones you want, that’s 75%. But how?! What’s the math?

We know the chance of getting heads on first coin is 50%. And getting heads on the second coin is 50%. We might be inclined to add the probabilities. That seems logical. But that gives us 100%. And we know that’s not right. What are we missing?

What we’re missing is that when we add 50% plus 50%, we’re counting one of the results twice.

The chance that the first coin comes up heads is 50%, right? Regardless of what the second coin does. That’s our starting point. What we need to add is all of the results in which the second coin comes up Heads that we didn’t ALREADY count. The first coin comes up heads in the HH and HT results. The second coin comes up heads in the HH and TH results. There’s one result that is included in both probabilities: HH.

Whenever we have two independent events – if those things can BOTH happen at the same time – we ADD the probabilities of each happening and then SUBTRACT the probability that they BOTH happen. Otherwise we end up doubling up.

So we end up with the chance of at least one heads among two coin tosses as being 50% + 50% – 25%. And that equals 75%.

Now, we can look back at our advantage situation. The probability of rolling an 8 or better on one die is the chance of rolling 8 or better on the first die PLUS the chance of rolling 8 or better on the second die MINUS the probability of rolling 8 or better on BOTH dice.

It’s easier to see this if we use a little math notation. In probability math, we use P to stand for the probability. And we’ll name our dice A and B.

P (A or B is 8 or better) = P (A is 8 or better) + P (B is 8 or better) – P (A and B are both 8 or better)

And we know that the chance of two things both happening at the same time just comes from multiplying the probabilities.

P (A and B are both 8 or better) = P (A is 8 or better) x P (B is 8 or better)

Putting it all together, we get:

P (A or B is 8 or better) =
P (A is 8 or better) + P (B is 8 or better) – [P (A is 8 or better) x P (B is 8 or better)]

And now we can just put in the numbers.

65% + 65% – (65% x 65%) = 87.75%

So, advantage improves the odds of rolling an 8 from 65% to 87.75%.

Now, even though I said that we generally worry about the probability of the roll you need on the die, it IS useful to understand how modifiers work when it comes to a d20. Because the chance of any given roll on a d20 is 5% (1/20), each increment on the die represents a 5% improvement in your odds. Put another way, imagine you’re trying to roll an 11 or better. Your chance of success is 50%. Now, imagine you have a +1 bonus. You only need a 10, now. Your chances have improved to 55%. In short, every +1 bonus is a 5% improvement.

And that tells us something interesting about advantage IN THIS CASE. The improvement from 65% to 87.75% is an improvement of 22.75%. That means it is the equivalent of +4 to +5. And that might imply Advantage has a lot of sway.

But here’s where things get tricky. Let’s do another one. Let’s assume you need a 17 on the die. I’m going to run through this quickly. Odds of a 17 or better on a d20 are 20%. So, the odds of a 17 on a d20 with advantage is 20% + 20% – (20% x 20%). And that equals 36%. Notice that’s only an improvement of 16%. And that’s about a +3.

In order to really understand the effects of advantage and disadvantage, you need to run out the results for every possible roll of the die. And that is because they aren’t LINEAR. And we’ll show why that’s important in a moment. For right now, though, if you’re curious, I slapped together a table for Advantage and Disadvantage.

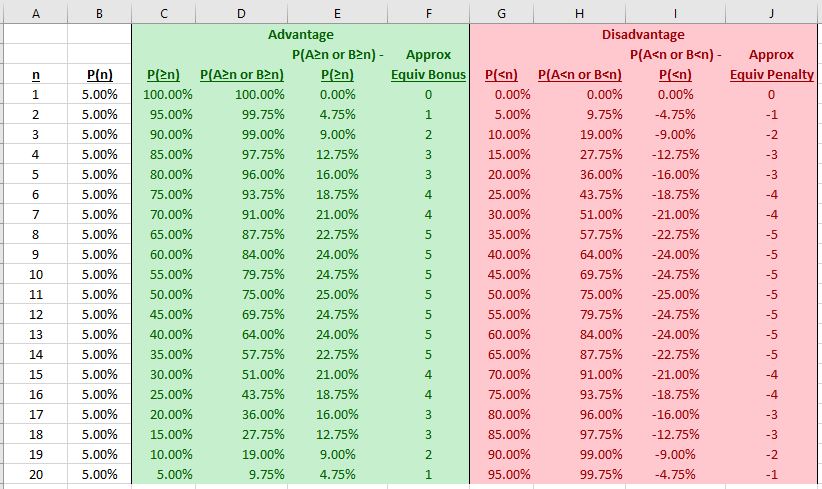
Now, you’ll probably notice I like to show ALL of my work and data. Column A is the result on the die. Column B is the chance of each result (5%). Column C is the cumulative result of that chance or better. Column G is the cumulative result of less than that number. And those two columns show us another thing about combining probabilities. When we want to know the chance of this OR that and there’s no way both this AND that can happen at the same time, we can just add the probabilities. On a d20, the chance of rolling a 1 is 5%. The chance of rolling a 2 is 5%. There is NO WAY to roll both a 1 and a 2 on the same die at the same time, so we can just add the probabilities. The chance of rolling a 1 OR a 2 is 10%. The chance of rolling a 1 or a 2 or a 3 is 15%. And so on. But if you’re savvy, you already knew that. We’re just counting the good outcomes and dividing by the total outcomes again.

It’s Column D and Column H that show the actual chances of succeeded or failing with Advantage or Disadvantage. Note that the Disadvantage section shows the chance of failure. Because I wanted to analyze the equivalent penalties. Columns E and I show how much your chance of success or failure changes with Advantage or Disadvantage. And Columns F and J show the equivalent bonus or penalty that Advantage or Disadvantage grants on average.

Basically, if you need a 10 on the die, Advantage is like having +5 and Disadvantage is like having a -5.

Now, I realize I worked out the Disadvantage side a little weirdly. It doesn’t show the chance of succeeding. It shows the chance of failing. And that’s because I wanted to know how much Disadvantage INCREASED the odds of failure and what modifier it would be equivalent to. But because you know you can invert probabilities by subtracting the probability from 100%, you can work out the odds of success if you want.

Now, you might notice that Advantage and Disadvantage behave a little weirdly. Notice that the biggest effects happen in the middle. If you need a result of 10 or thereabout, Advantage and Disadvantage have big effects. If you need a result of a 2 or a 19, they have very small effects. Why? Well, we can answer that by looking at another problem. What if you replaced the 1d20 in Pathfinder and D&D with 3d6?

What if You Used 3d6?

And that brings us around to another question we can use math to answer now. And this one will illustrate what we mean by linearity. And it will also introduce a very important concept: the standard deviation.

Let’s say you are sick of using the d20. Instead, you want to replace it with 3d6. 3d6 gives results between 3 and 18. So, it tightens things up a little and eliminates the extremes, right? Yes. It does. But it also has another effect.

Essentially, the d20 is a linear probability. Every result has the same chance of occurring, 5%. But that isn’t the case with 3d6. 3d6 is a little weird. To see why, we have to look at the results we can roll.

Let’s say you get a 3 on 3d6. What did the dice look like? Well, there’s only one way to roll 3 on 3d6. You rolled three 1s. That’s the only combination of rolls that adds up to 3. But what if you rolled a 4? That gets a little funnier. Obviously, I rolled a 2, a 1, and a 1. But there’s three different dice. Let’s call them A, B, and C. Die A could have been a 2 and then B and C are 1s. Or Die B could have been the 2, leaving A and C as 1s. Or C could have been the 2 and A and B are the 1s. There’s actually three different unique combinations of rolls that add up to 4. And this continues. There’s 6 different ways to make a 5. There’s 27 different ways to make a 10. And 27 different ways to make an 11. And who knows how many different total combinations there even are.

Well, I do. It’s 216. There’s 216 possible combinations of rolls for 3d6. Basically, there’s 6 outcomes on the first die, 6 on the second, and 6 on the third. 6 times 6 times 6 is 216.

That means, with only 1 combination out of 216 to roll a 3, that’s only a 0.46% chance. Yes. Less than half a percent. With 27 combinations out of 216 to roll a 10, you’re 12.50% likely to roll a 10. You see what’s happening here? And the same happens at the high end. The only way to roll an 18 is to roll three 6s. That means there’s only 1 combination out of 216 to roll an 18. But there’s 27 combinations that create an 11.

If we put all the odds of both the d20 and 3d6 on a table, you can see the difference.

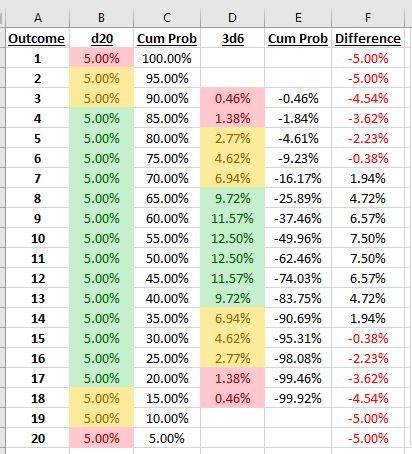
The chance of rolling any given result on a d20 is 5%. And the cumulative chance of rolling that number or above goes up by 5 percentage points each time. But the odds of rolling a given number on 3d6 increases as you get close to 10 and then it starts to decrease again. The cumulative odds of rolling a given number or above swing wildly. And if we show the differences between the probabilities, you can see that, when rolling 3d6, you’re MORE likely to roll between 7 and 14 and LESS likely to roll below 7 or above 14.

And if we put the results on a graph, we can those results more visually.

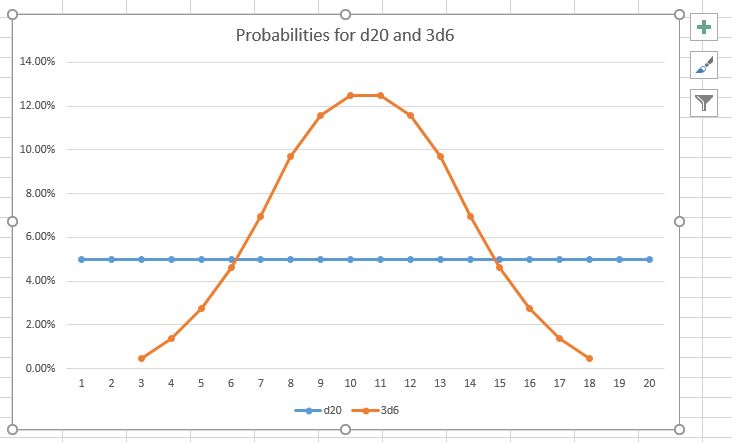
The 3d6 probabilities clump up in the middle. The d20 probabilities stay nice and flat. And that’s why we call the d20 a LINEAR probability curve. The 3d6 curve is what we call a NORMAL DISTRIBUTION or BELL CURVE. And that has some very important implications.

The implications come down to something called “standard deviation.” Standard deviation is a measure of how “swingy” or “spread out” the results are. More accurately, it tells us how likely any given result is to deviate from the average, the mean, the expected result.

See, what’s interesting about both the d20 and 3d6 results is that they have exactly the same average: 10.5. But that doesn’t tell us the whole story. Because the d20 results are fairly spread out and the 3d6 results are clumped up. Standard deviation establishes a range. For example, the standard deviation for 3d6 is 2.96. What does that mean? It means that a lot of the time, when you roll 3d6, you’re going to get a result that is within about 3 of 10.5. Basically, from 7.5 to 13.5. We call that “being within one standard deviation.” You can also be within two standard deviations. That’s a number from 4.5 to 17.5.

Mathematicians refer to the “68-95-99.7 rule.” 68% of all the results lie within one standard deviation of the average. 95% of all the results lie within two standard deviations. And 99.7% of all the results lie within three standard deviations. If you roll 3d6, 68% of the time, you’re going to get a number between 7 and 13. 95% of the time, the result will be between 4 and 17.

The bigger the standard deviation, the more spread out the data is. The standard deviation for rolling 1d20 is 5.77. That means that 68% of all d20 rolls will end up between 4 and 16.

I’ve highlighted the standard deviations (roughly, and rounded off) on that spreadsheet.

Green results lie within one standard deviation of the average (10.5). Yellow lie within two standard deviations of the average. Red lie within three standard deviations. Hopefully, that makes it easy to see.

Why does this happen with 3d6? And why do the results clump up with Advantage and Disadvantage? Well, it actually has to do with the Law of Averages. Recall that, if we do a random thing, the outcome is random. It could be anything. And probability doesn’t have a lot to say about a single roll. Not much that’s interesting anyway. But when we start rolling lots and lots of times, things to tend to skew toward the average.

When you roll 2d20, you’re rolling twice as many rolls. When you roll 3d6, you’re rolling three times as many rolls. So, your results ON EACH DIE tend to skew toward the average. In effect, you’re getting to the Law of Averages twice as fast or three times as fast. Now, each individual roll is still random and you might roll a 3 or 18 or 12, but when we consider the overall probability, results start to clump up in the middle.

By the way, this is why casinos don’t care if someone wins the jackpot. They’ve rigged the system so that, on average, they are making a little bit of money on every roll. As long as lots of people play lots of games every day, the occasionally extreme result (jackpot) doesn’t even phase them.

Knowing what we know now, what IS the impact of swapping out a d20 for 3d6? Well, obviously, it skews the probabilities toward the middle (even if it doesn’t quite change the average result). But you can take that into consideration. Notice I ran out the cumulative probabilities so you can see how likely it is to roll, say, 7 or above. And it does iron out the extreme results. But there’s a couple of interesting things to note.

First of all, notice that a critical hit on an 18 is extremely unlikely. If you want to maintain the critical hit chance (5%) of a d20, you probably want to have crits happen on a roll from 16 to 18. If you don’t mind cutting crits down to 3%, you can say any triple roll is a crit (triple 1s, triple 2s, etc.). There’s 6 combinations of triple rolls out of 216, and that’s roughly 2.8%.

Second of all, notice that modifiers become a bit weirder though. With a d20, we know that every +1 increases the chance of success by 5% (technically, 5 percentage points – which I only mention so actual mathematicians won’t point out the subtle difference). But, with 3d6, the value of a +1 changes depending on the roll needed. For example, if you need an 11, a +1 bonus increases your odds from 37% to 50%. But if you need a 15, a +1 bonus only increases your odds from 5% to 9%. That means modifiers are most valuable for average tasks. For extremely easy and extremely difficult tasks, bonuses and penalties don’t have much impact.

Of course, maybe that’s precisely what you want.

Daunting Math

Now, the thing about probability math is that it can get very daunting very quickly. One of the reasons I didn’t cover the calculation of standard deviations is because you have to do a lot of repetitive math. Basically, you need a weighted average and then you need to subtract that from each result, weighted by probability. Then, you square all those results, add them all up, and take the square root. At that point, it’s a hell of a lot easier to let a spreadsheet do it. In fact, I didn’t even bother. When I did the d20 and 3d6 thing, I eyeballed it. I highlighted the 65% range and the 95% range by eye.

Take, for example, the problem of rolling 4d6 and dropping the lowest to generate ability scores. However, you slice up that problem, it involves a HEAVY spreadsheet or a lot of math. Enumerating the results is pretty tricky. There are 1,296 combinations of rolls, from 1, 1, 1, 1 to 6, 6, 6, 6. And you have to translate each of them to an actual result. For example, 2, 3, 4, 6 translates to 13 because you drop the lowest die. It takes a long time to count them all up.

And this problem is tricky because it’s hard to even figure out the average. Things get skewed and wonky because of the die dropping. Let me give you one example. When you roll 3d6, the results are symmetrical. There’s only one combination that leads to a 3 (1,1,1) and only one that leads to 18 (6,6,6). But the results aren’t symmetrical if you drop the lowest die. The only way to get a 3 is 1,1,1,1, but 6,6,6,1; 6,6,6,2; 6,6,6,3; 6,6,6,4; 6,6,6,5; and 6,6,6,6; all yield 18 because of dropping the lowest die. An 18 is six times as likely as a 3.

There is actually a very powerful tool on the web that I located VERY LATE in building this article. WHICH IS WHY I DID MOST OF MY OWN MATH. But even I was not dumb enough to do the 4d6 problem myself. I COULD. I could build that f$&%ing spreadsheet. But it would take ages.

If you visit http://anydice.com/, you can input any combination of dice you can think of and see the probabilities for each result, the average, and the standard deviation. If you want, for example, to check any of my probability math, you can put the following commands into AnyDice:

output 1d20
output 3d6
output [highest 1 of 2d20]
output [lowest 1 of 2d20]
output [highest 3 of 4d6]

It’s that last one, we’re concerned with. By the way, if you add the command ‘named “Name”’, AnyDice will give your data nice headers. For example:

Now, I’ve taken the data from the “3d6” and “4d6 drop lowest” and put them in a spreadsheet and a pretty graph because I like playing with Excel even though it is a f$&%ing nightmare to get it to JUST MAKE A SIMPLE F$&%ING GRAPH OF HIGHLIGHTED DATA! And that should help you see how rolling 4d6 and dropping the lowest skews the results.

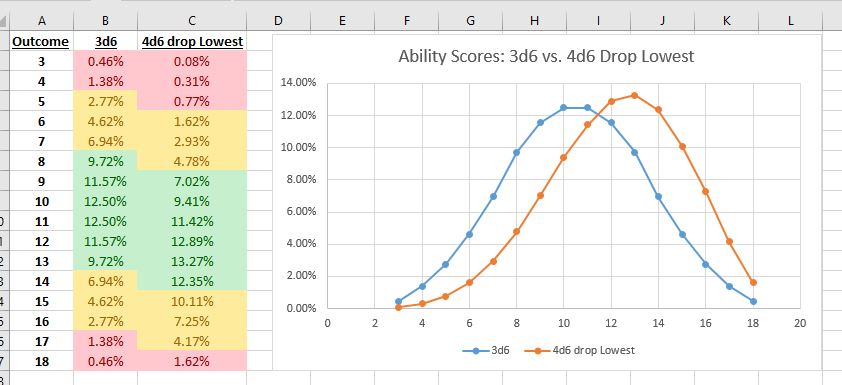
The average of 4d6 when you drop the lowest die is 12.24 and the standard deviation is 2.85. The average of 3d6 is 10.5 and the standard deviation is 2.96. The 4d6 method is slightly tighter, but not much (because the standard deviation is lower), but it does shift the average up by almost two. The end result is that we expect two thirds of someone’s ability scores to land between 9 and 14. We expect the last two fall between about 7 and 17.

Now, does that sound familiar. Yeah, it’s close to the “standard array” that D&D and Pathfinder adopted: 15, 14, 13, 12, 10, 8. Now, if we look closely at the numbers, we can guess that, if you choose to ROLL for ability scores, there’s only about a 10% chance you will do better than the standard array, but fortunately, there’s only a 5% chance you’ll do worse. Very, very roughly.

Given that, is it even WORTH rolling ability scores? Probably not. But some players still won’t let that bulls$&% go.

Final Note: Don’t Go Too Crazy

Okay, obviously, I LOVE math and spreadsheets and graphs and rigorous analysis. And I’m not going to apologize. And obviously, D&D’s designers did look at SOME of this s$&% when creating 5th Edition (and 4th Edition). 3rd Edition is a little wonkier. But I will point out that the 1st Edition AD&D DMG did actually have a discussion about probability, linear curves, bell curves, and ability score generation pretty early on.

But, here’s the thing: it’s possible to go TOO crazy with stuff. Like I do. The truth of the matter is that some game designers are really well versed in this s$&%, but I’m pretty sure a lot of them aren’t. And moreover, if you look at the mechanics of some systems, it’s pretty clear no one is doing this sort of math just based on what a pain in the a$&% the math would be or based on the end result. I know Gygax did. But Gygax was a f$&%ing insurance actuary. He loved statistics. And I do to. So I sympathize.

The thing is, at the end of the day, what matters is how things feel. The D&D designers have done focus testing, for example, to figure out how often you need to succeed on a die roll for most folks to feel like things are fair and fun. At least, they claim they have, and I believe them. The math just let them figure out how to bake that probability into the game. But there’s a certain point where math rigor doesn’t get you anything.

I think every GM should understand how dice work and understand some of the basics of probability. At least enough to understand, conceptual, how f$&%ing with the mechanics changes the system. But none of that math is a substitute for doing what feels good. What feels fun. Or engaging. So don’t let the numbers keep you from experimenting. And don’t try to build perfect systems. Part of the reason we LIKE randomness in games is because we like unexpected and crazy results. Sometimes, we want things to be a little crazy.

Anyway… for all of you who read all of that s$&%, I’m truly sorry. Next week, I’ll talk about NPC villains or some s$&% like that. I promise.